Part 1
Maximum Turns Formula for Braided Rubber Motors
by Gary Hinze, San Jose, California
24 March, 2006
A rubber strip motor is said to be braided when the strip of rubber is twisted before tying the ends together to form a loop. How does the number of braiding turns affect the maximum number of turns that can be put into the motor? I propose the following formula:
The maximum number of turns that may be put into a braided motor is the maximum number of turns that may be put into the unbraided motor, less 0.3536 times the number of braiding turns.
The derivation of this formula requires some explanation.
In order to fit a long motor into a short space, and to prevent bunching and flopping around of the loose motor after unwinding, which causes CG shifts, modelers “braid” the motor by twisting the halves before doubling and tying the ends together. Methods of doing this are well discussed elsewhere:
http://www.aalmps.com/braid.htm
http://www.gryffinaero.com/models/ffpages/tips/motorbraid.html
The question addressed here is the reduction in the maximum number of turns that may be put into the finished braided motor compared with the maximum number of turns that may be put into an unbraided motor of the same dimensions.
It has often been said that the maximum energy that may be stored in rubber by twisting is equal to that stored by stretching. Extending this idea, we may say that the total energy that may be put into a braided motor is equal to what may be put into it by winding without braiding. This is reasonable, since the stresses in the rubber twist and knot rubber in such a way as to distribute the stresses as uniformly as possible. After a few turns, the stresses imposed by the braiding will blend in with the stresses of winding. If the stretching is uniformly applied to the rubber molecules, there will be a certain amount of energy that may be stored in a given mass of rubber, no matter what geometric form the stretching takes. This suggests a simple method of developing a formula for estimating the maximum turns that may be put into a braided motor.
We are familiar with torque versus remaining turns curves. Cumulatively adding up the energy each turn adds to the motor produces an energy versus turns curve. Each turn adds energy equal to two pi times the torque at the respective turn number.
The derivation of the braiding turns formula is based on the comparison of the energy curve for the prebraided rubber of twice the length and half the cross section of the final motor, before doubling it to form the finished motor, with the energy curve of the finished unbraided doubled motor. Consider the simplest case where a single strand is doubled over to make the full motor. Consider the energy versus turns remaining curve for the single strand and the energy versus turns remaining curve for the double strand motor of half the original strand length and twice the cross section. Both curves may be plotted on the same graph. Both curves have the same maximum, since both motors weigh the same and have the same energy storage capacity. Look at the first curve for the energy corresponding to the number of braiding turns put into the single strand. Look at the number of turns that corresponds to that same amount of energy on the curve for the doubled motor. Deduct that number of turns from the maximum turns for the double strand motor. That is the maximum number of turns that may be put into the braided motor.
This leads to the development of a simple formula for maximum turns in a braided motor. Plot both energy curves backwards on the same axis. In other words, plot the energy remaining versus unwinding turns curves on the same pair of axis. Both start out with the same amount of energy, and energy decreases as the motor unwinds. The number of unwinding turns is inversely proportional to the square root of of the motor cross section and proportional to its length. The single strand has twice the length and half the cross section of the double strand motor. So it has twice the square root of two (2.8284) times the maximum turns of the double strand motor. The number of unwinding turns at any energy level, represented by a horizontal line through that energy level, is in the same ratio between the two curves, since the curves are proportional to each other. Therefore the number of unwinding turns in the double strand motor is equal to the number of unwinding turns in the single strand motor with the same energy, divided by twice the square root of two. Unwinding turns and winding turns always add up to the maximum turns that can be put into the motor. This leads directly to a formula for maximum turns in a braided motor. The maximum turns that may be put into a braided motor is equal to the maximum number of turns that may be put into the single strand, less the number of braiding turns put into the strand, all divided by twice the square root of two.
When Ts is the maximum number of turns that may be put into the single strand and Tb is the number of braiding turns put into the single strand, the formula for the maximum number of turns that may be put into the braided motor is Tm = (Ts-Tb)/2xSQRT(2) = 0.3536x(Ts-Tb).
It happens that the maximum number of turns that may be put into the unbraided double strand motor is related to the maximum number of turns that can be put into the single strand. Td = Ts/2xSQRT(2), where Td is the maximum number of turns that can be put into the unbraided double strand motor. This means the formula may be expressed as Tm = Ts/2xSQRT(2) – Tb/2xSQRT(2) = Td-Tb/2xSQRT(2) = Td-0.3536xTb.
Since 1/(2xSQRT(2)) = 1/2.8284 = 0.3536.
In words, the maximum number of turns that may be put into a braided motor is the maximum number of turns that may be put into the unbraided motor, less 0.3536 times the number of braiding turns.
QED
John Barker’s proposed formula, that the maximum number of turns that may be put into a braided motor equals the maximum number of turns that may be put into the unbraided motor, less half the number of braiding turns, is more conservative. It also means you will not be utilizing the high proportion of energy in the top turns. You will be losing a lot more energy than just that taken up by the braiding turns.
No torque meter tests of braided motors are necessary. The formula works for any torque curve. It is a matter of geometry, not a material property.
This also tells you how much energy is sacrificed by braiding. The remaining unwinding energy that corresponds to the number of braiding turns that are put into the single strand is unavailable for flight. It must be deducted from the total energy that may be recovered from the motor. Energy equates to time aloft. The time lost in still air due to energy unavailable due to braiding is equal to the lost energy divided by the product of the weight of the aircraft and the sinking speed of the aircraft. Fortunately there is very little energy in the first few turns of the half cross section motor.
Hank Sperzel referred to Bill Winter’s “Model Aircraft Plan Book”, page 71, section on Lanzo’s 1946 Wakefield “Defender” for an example of a braided motor. This is interesting enough that I will quote the relevant part. It provides an example for calculation of turns and energy lost to braiding. It says:
“The clever designer manages an extreme rubber length — far longer than the distance between the two rubber hooks — and a good folding propeller uses those precious rubber turns efficiently, besides reducing the drag in the glide. Lanzo, for example, almost doubles his rubber length, using a 48-inch long motor of 24 strands (not loops) of 3/16” flat brown rubber. To keep tension on such a long rubber motor as it winds down, so that it won’t sag and shift within the fuselage to cause diving or stalling, most builders prefer “braiding” the motor. This means that they separate the rubber into two groups of strands, by doubling the motor around a post, then wind each group about fifty turns, finally bringing together again both groups of strands. Although this keeps the rubber taught between the hooks, it is possible to put a tremendous number of turns into such a motor. This is known as the White rope tensioner. (The White rope type of tensioner is named after Mr. H. White, of England, who invented it. — The Editor).”
Rope is made by tightly twisting individual strands and allowing them to intertwine. What we do might more appropriately be called “roping”, but the term “braiding” has been in widespread use for many decades and so has become accepted usage.
To calculate the maximum number of turns that may be put into this braided motor, I look to Charles Hampson Grant’s extensive tests of flat brown rubber of this vintage, published in “Aero Science of Free Flight”, originally published in “Model Airplane Design and Theory of Flight”, 1941-1944. This rubber strip was 1/30” thick and had a turns coefficient of about 8. The cross section of the final motor is 24×3/16×1/30 = 0.15 square inch. The maximum number of turns per inch that may be put into the motor is 8/SQRT(0.15) = 20.66, so 991 turns could be put into the 48” motor. Fifty turns were put into each end of the split motor, for a total of 100 braiding turns. The formula says the maximum number of turns that can be put into the braided motor equals the maximum turns that can be put into the unbraided motor, less 0.3536 times the number of braiding turns, in this case, 991-35 = 956 turns. That is a loss of 3.6% off the maximum unbraided turns. The energy loss is in the braiding turns which go into the half section motor, so it is minor, estimated as less than about 1/2%.
I think Bill Henn for posing this problem on FFML, Fred Rash for providing a copy of Hewitt Philips NFFS Digest article “Generalized Energy Curves for Rubber Motors”, Hank Sperzel for the Lanzo “Defender” article and John Barker for suggesting I take a simpler approach than trying to figure out the breaking stress on a knotted, braided motor.
Part 2
John Barker’s approach captures something that I overlooked. You can see it here:
https://freeflight.org/Library/TechLibrary/BraidingLostTurns.pdf
John considers the relationship between braiding turns put into the original motor and the number of braids that results when the motor is doubled. He also points out something I overlooked; that when you wind a braided motor, the braids come out and at some point you have the original motor, bent in half, with the total braiding turns in it. At that point, the original braiding turns are in the full loop and the initial energy is stored in the motor. That means this is not the place to start counting turns. We must start counting with the braids, which correspond to a slightly lower energy preloading. This requires confirmation of the relationship between braiding turns and braids.
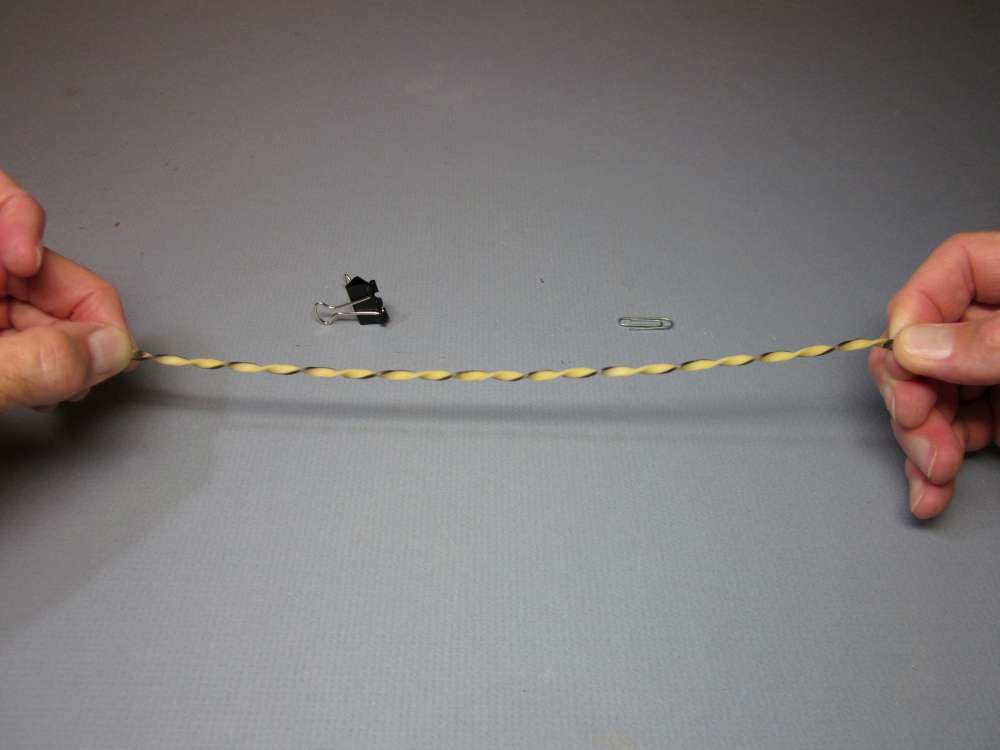
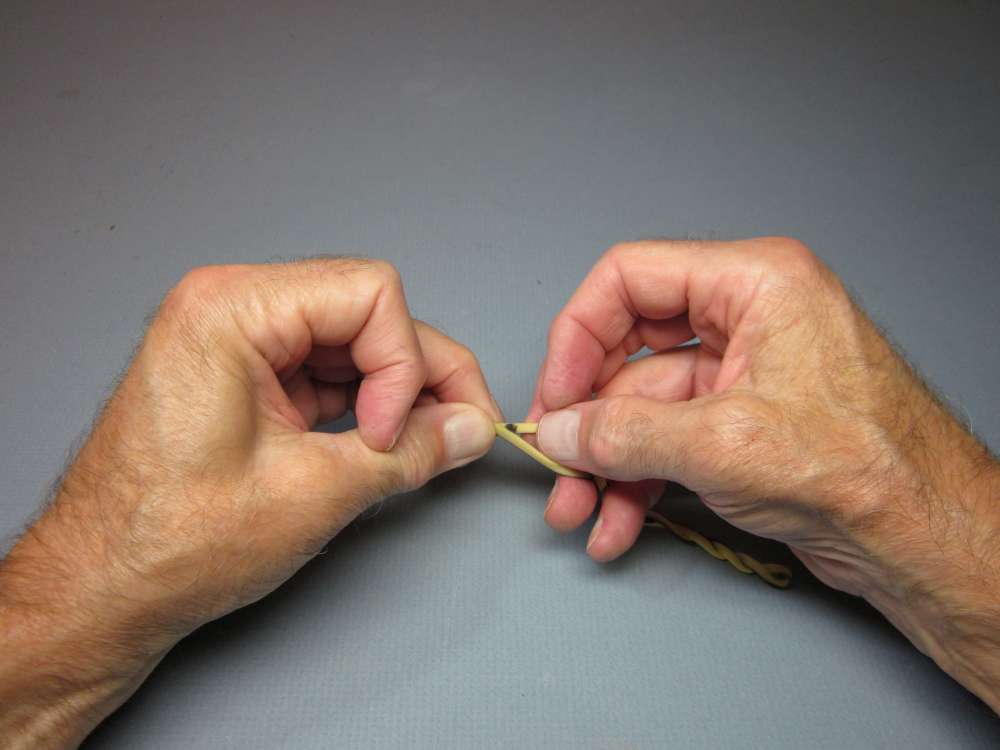
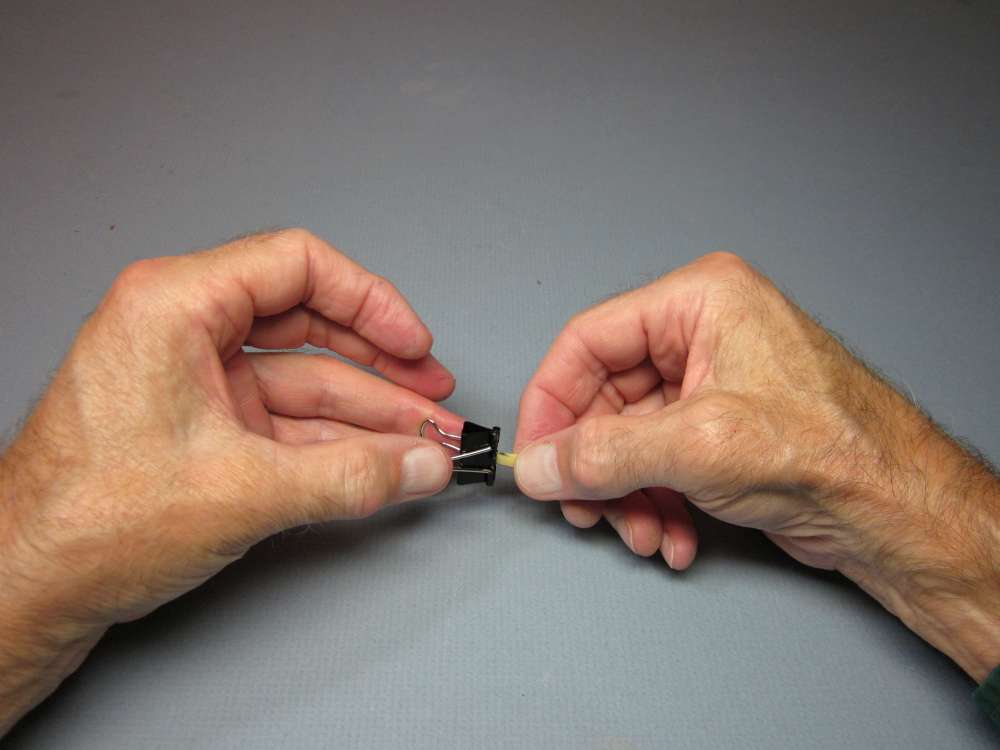
This led me to take another look at my twisting tests. I had used unlubed rubber strip, twisted it and counted twists and braids. John had given good reason to believe the number of twists should be four times the number of braids. I had found ratios from 2.5 to 5.0. I decided to redo my tests, with well lubed motors, carefully braided.
In a braided 2-strand rubber motor, let
| Rubber Motor Turns and Braids | ||||||
| T | B | S | 2B+2S | T/B | Note | |
| 8 | 3 | 1 | 8 | 2.67 | Not fully braided | |
| 12 | 4 | 2 | 12 | 3.00 | Not fully braided | |
| 16 | 5 | 3 | 16 | 3.20 | Full but loose braids | |
| 20 | 6 | 4 | 20 | 3.33 | Full braid | |
| 24 | 7 | 5 | 24 | 3.43 | Full braid | |
| 28 | 8 | 6 | 28 | 3.50 | Tight braid | |
The columns for B and S are very regular. The relationships are linear. You can see that B = T/4 + 1 and S = T/4 – 1. (Division takes precedence over addition and subtraction.) Also, B = S + 2, 2B+2S = T and T/B = 4T/(T+4). Note that T/B approaches a limit of 4 as T increases “to infinity”, it should never equal 4. That John and I got values of 4 or more suggests that friction kept some of the twists from becoming braids.
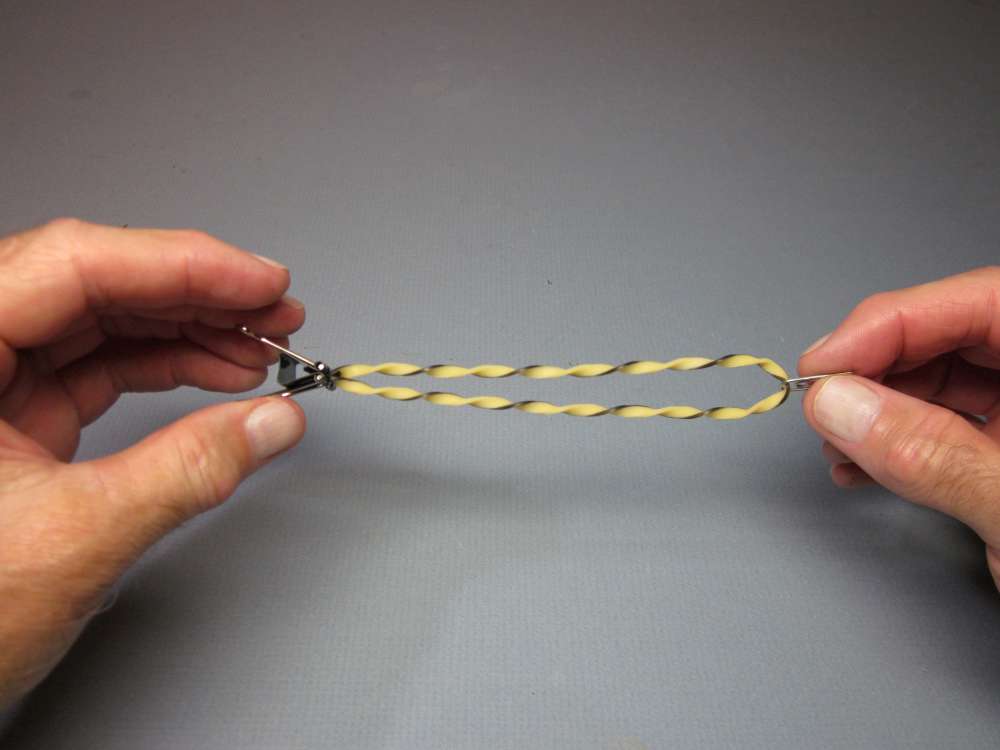
I wonder if there is anywhere that I can get a copy of this information.
This is it, right here.